(Untitled)
This is why I hate doing the math to construct a soaker from scratch, and am trying to write up the pattern so I never have to do it again.
I usually do trial and error to a point and then jimmy some figures so they work. This time I'm trying to be more methodical about it so I've come up with a system of equations to solve.
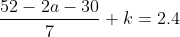
( Read more... )
I usually do trial and error to a point and then jimmy some figures so they work. This time I'm trying to be more methodical about it so I've come up with a system of equations to solve.
( Read more... )
Comments 7
Reply
Reply
Reply
Reply
Reply
(The comment has been removed)
I'm knitting a soaker, and at the point where the legs split for front and back. I'm balancing the need to make the leg opening fit the thigh and the length fit the rise measurement. a is the number of stitches to bind off at each side. k is the length in inches to knit even after finishing the decreases to the crotch width.
*head explodey*
I think I need to change some of my base assumptions, like the rise at which I'm going to start the split and decreases...
Reply
Reply
Leave a comment